問題 6-1 の解答例

はてなブログ：「ファインマンさんの肩に乗って晴耕雨読の日々」に書いていた記事を今日から順にこちらに移していこう．先ずはファインマン経路積分の第6章の問題をまとめて移してしまう．

Problem 6-1
Suppose the potential can be written as $U + V$ , where $V$ is small but $U$ is large. Suppose further that the kernel for motion in the potential of $U$ alone can be worked out ( for example, $U$ might be quadratic in $x$ and independent of time ). Show that the motion in the complete potential $U + V$ is described by Eqs. (6-4), (6-11), (6-13), and (6-14) with $K_{0}$ replaced by $K_{U}$ , where $K_{U}$ is the kernel for motion in the potential $U$ alone. Thus we can consider $V$ as a perturbation on the potential $U$ . We can say that $(- i / ℏ) V$ is the amplitude to be scattered by the perturbing part of the potential ( per unit volume and per unit time ). $K_{U}$ is the amplitude for the motion in the system in the unperturbed potential $U$ .

この場合, 式 (6.1) に相当する式は次となる：

\begin{aligned} K_{U + V} (b, a) & = \int_{a}^{b} D x (t) \exp [\frac{i}{ℏ} \int_{t_{a}}^{t_{b}} d t {\frac{m}{2} {\dot{x}}^{2} - U (x, t) - V (x, t)}] \\ (1) & = \int_{a}^{b} D x (t) \exp [\frac{i}{ℏ} \int_{t_{a}}^{t_{b}} d t {\frac{m}{2} {\dot{x}}^{2} - U (x, t)}] \exp [- \frac{i}{ℏ} \int_{t_{a}}^{t_{b}} d t V (x, t)] \end{aligned}

今度は, ポテンシャル

U + V

の内の

V

だけを「ポテンシャルの小さい部分」とすることで, やはり式 (6.3) と同様な級数展開が出来る：

\begin{array}{r} (2) & \exp [- \frac{i}{ℏ} \int_{t_{a}}^{t_{b}} d t V (x, t)] = 1 - \frac{i}{ℏ} \int_{t_{a}}^{t_{b}} d t V (x, t) + \frac{1}{2!} {(\frac{i}{ℏ})}^{2} {[\int_{t_{a}}^{t_{b}} d t V (x, t)]}^{2} + \dots \end{array}

これを式 (1) に代入すれば, 式 (6.4) に相当する式として次が得られる：

\begin{aligned} K_{U + V} (b, a) & = \int_{a}^{b} D x (t) \exp {\frac{i}{ℏ} \int_{t_{a}}^{t_{b}} d t [\frac{m}{2} {\dot{x}}^{2} - U (x, t)]} \\ \times [1 - \frac{i}{ℏ} \int_{t_{a}}^{t_{b}} d t V (x, t) + \frac{1}{2!} {(\frac{i}{ℏ})}^{2} {[\int_{t_{a}}^{t_{b}} d t V (x, t)]}^{2} + \dots] \\ (3) & = K_{U} (b, a) + K^{(1)} (b, a) + K^{(2)} (b, a) + \dots \end{aligned}

ただし式 (6.5) の

K_{0} (b, a)

に相当するのは, 次の

K_{U} (b, a)

となる：

\begin{matrix} (4) & K_{U} (b, a) = \int_{a}^{b} D x (t) \exp {\frac{i}{ℏ} \int_{t_{a}}^{t_{b}} d t [\frac{m}{2} {\dot{x}}^{2} - U (x, t)]} \end{matrix}

また

K^{(1)} (b, a)

や

K^{(2)} (b, a)

は次となる：

\begin{aligned} (5) & K^{(1)} (b, a) & = - \frac{i}{ℏ} \int_{a}^{b} D x (t) \exp {\frac{i}{ℏ} \int_{t_{a}}^{t_{b}} d t [\frac{m {\dot{x}}^{2}}{2} - U (x, t)]} \int_{t_{a}}^{t_{b}} d s V [x (s), s], \\ K^{(2)} (b, a) & = - \frac{1}{2 ℏ^{2}} \int_{a}^{b} D x (t) \exp {\frac{i}{ℏ} \int_{t_{a}}^{t_{b}} d t [\frac{m {\dot{x}}^{2}}{2} - U (x, t)]} \\ (6) & \times \int_{t_{a}}^{t_{b}} d s V [x (s), s] \int_{t_{a}}^{t_{b}} d s^{‘} V [x (s^{‘}), s^{‘}] \end{aligned}

そしてポテンシャル

U + V

による散乱を, 摂動ポテンシャル

V

による散乱と解釈するには, 式 (6.12) における自由粒子核

K_{0} (b, a)

を, ポテンシャル

U

の下での粒子核

K_{U} (b, a)

で置き換えればよい：

\begin{matrix} (7) & K_{U} (b, c) [- \frac{i}{ℏ} V (c) d x_{c} d t_{c}] K_{U} (c, a) \end{matrix}

ただし

V (c)

は点

c

におけるポテンシャルの摂動部分である (下図を参照)．よって, 式 (6.11) や式 (6.13), 式 (6.14) に相当する式は次となる：

\begin{aligned} (8) & K_{U + V} (b, a) & = K_{U} (b, a) + K^{(1)} (b, a) + K^{(2)} (b, a) + \dots, \\ (9) & K^{(1)} (b, a) & = - \frac{i}{ℏ} \int_{t_{a}}^{t_{b}} d t_{c} \int_{- \infty}^{\infty} d x_{c} K_{U} (b, c) V (c) K_{U} (c, a), \\ (10) & K^{(2)} (b, a) & = {(- \frac{i}{ℏ})}^{2} \int d τ_{c} \int d τ_{d} K_{U} (b, c) V (c) K_{U} (c, d) V (d) K_{U} (d, a), \\ K^{(i)} (b, a) & = 0, (t_{b} < t_{a}), i = 1, 2, 3, \dots \end{aligned}

ただし

d τ = d x d t

である．

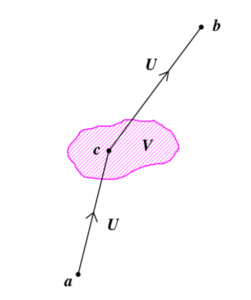
図 1. この場合には, 自由粒子でなく, ポテンシャル $U$ の下で運動している粒子が, 点 $c$ で小さなポテンシャル $V$ による散乱を受け, その後また同様にポテンシャル $U$ の下で運動して行くと考える．

月	火	水	木	金	土	日
	1	2	3	4	5	6
7	8	9	10	11	12	13
14	15	16	17	18	19	20
21	22	23	24	25	26	27
28	29	30